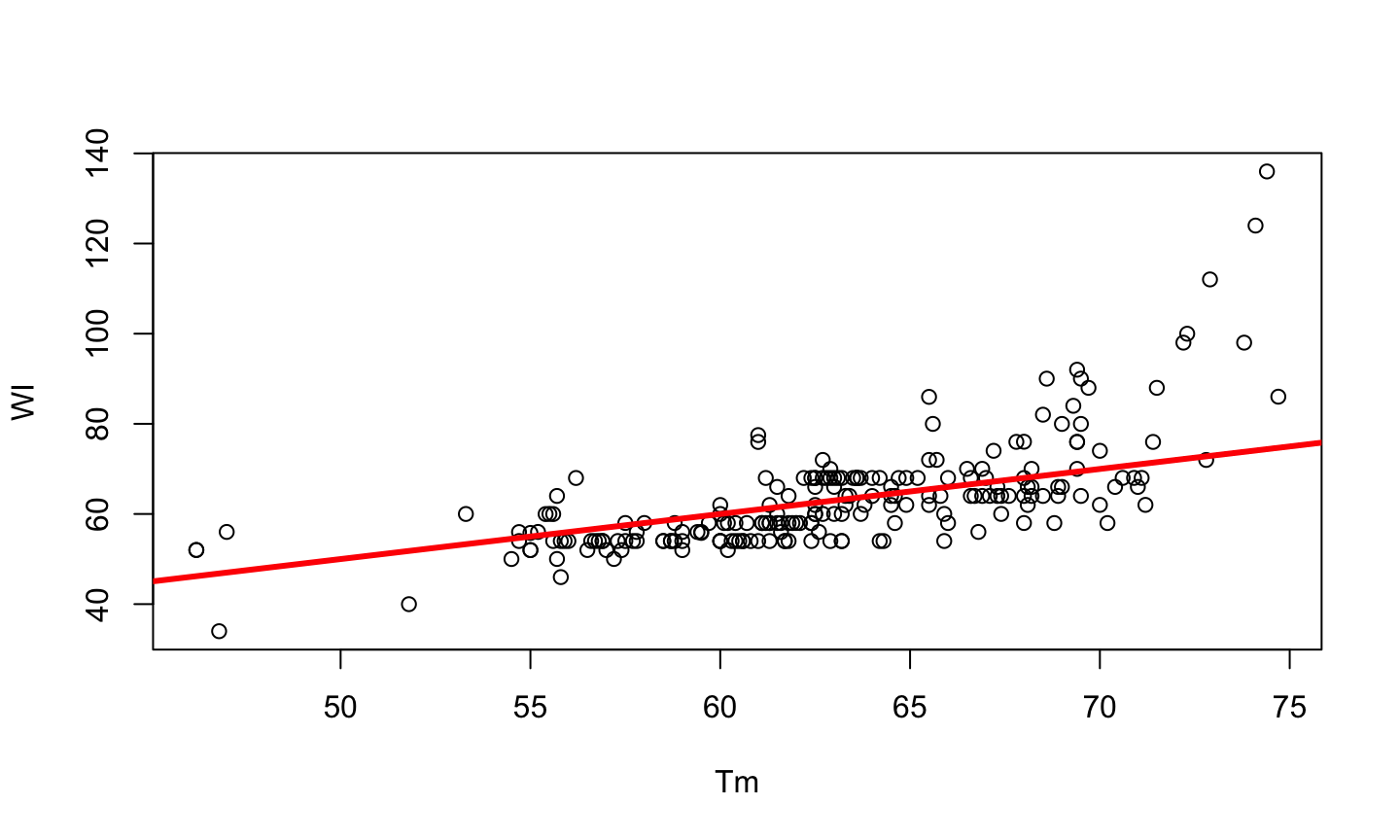
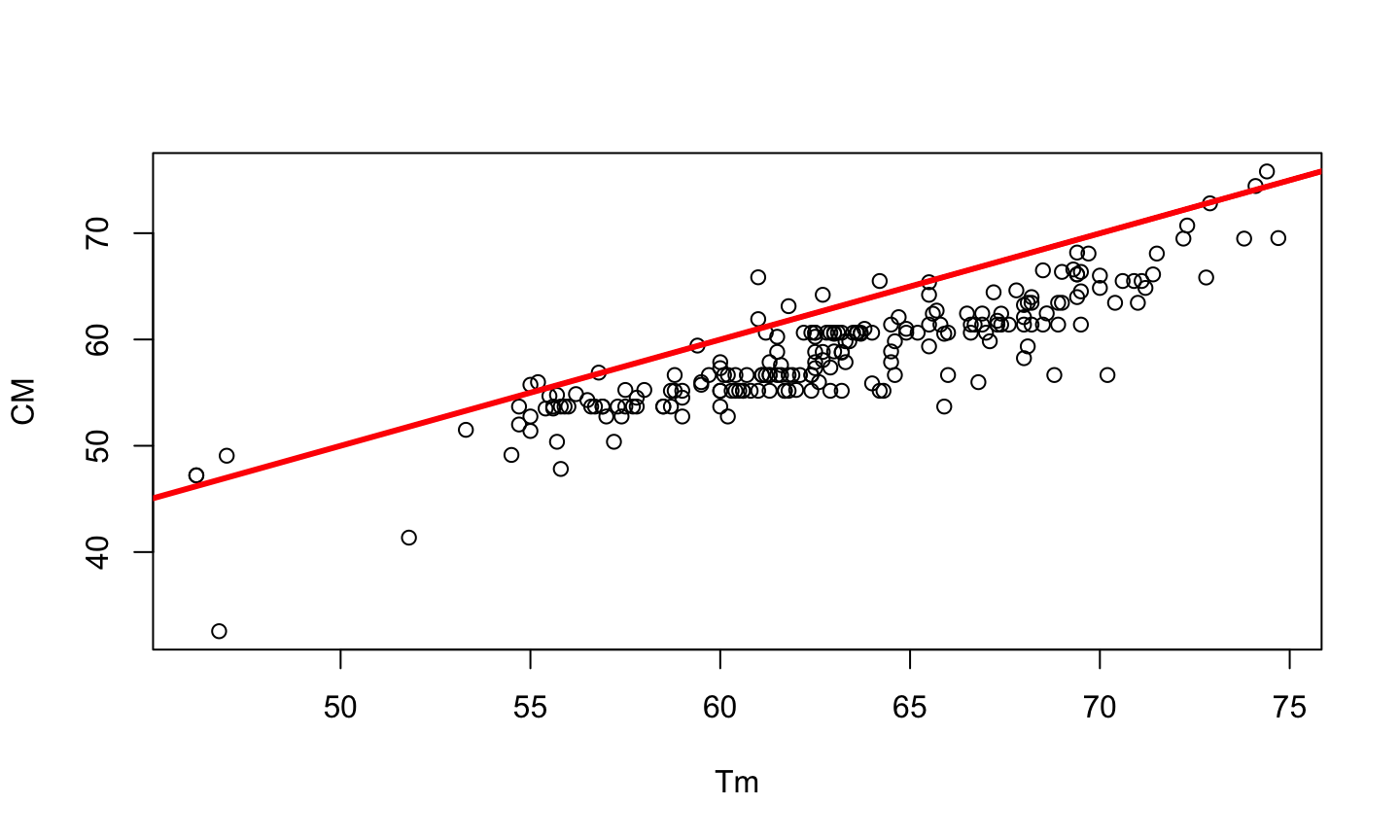
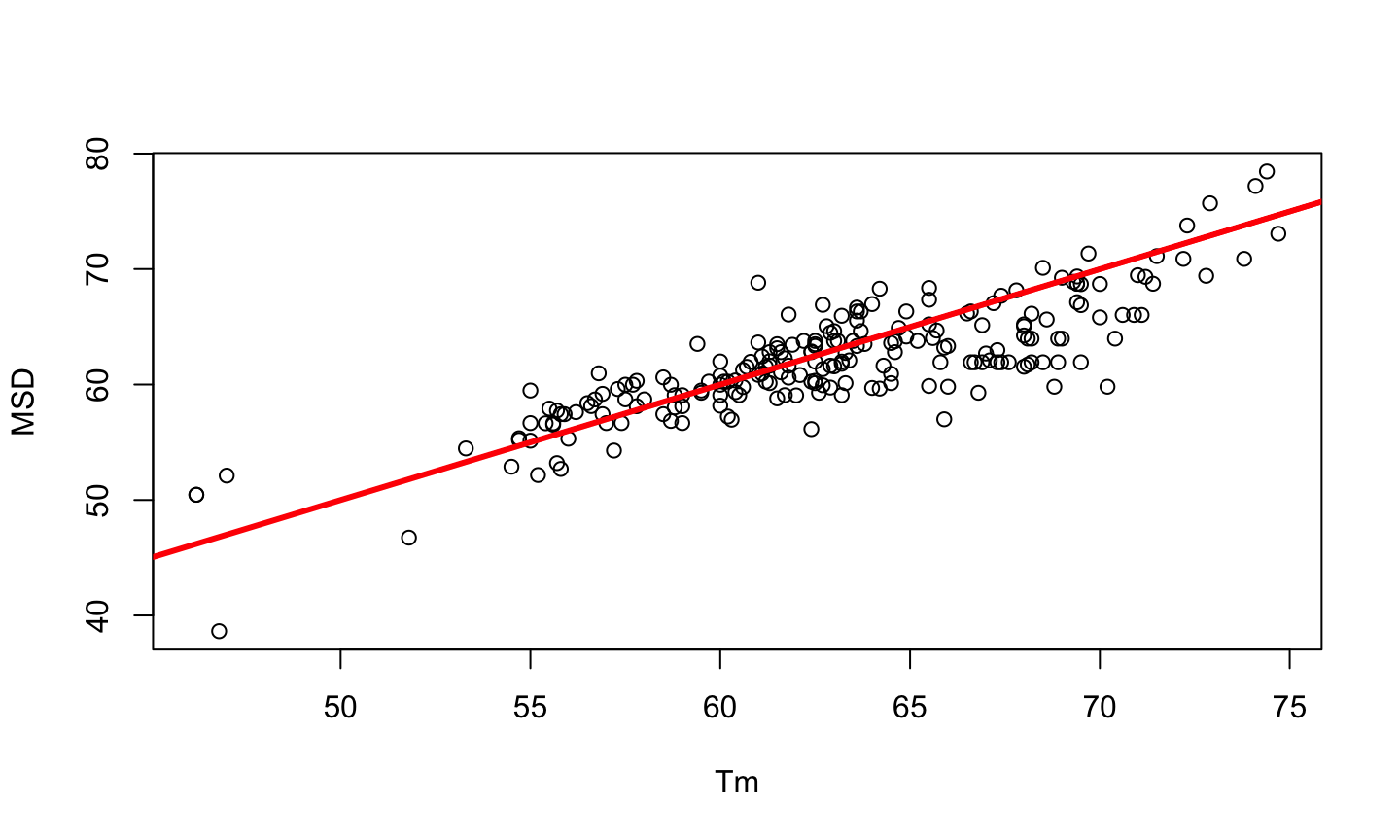
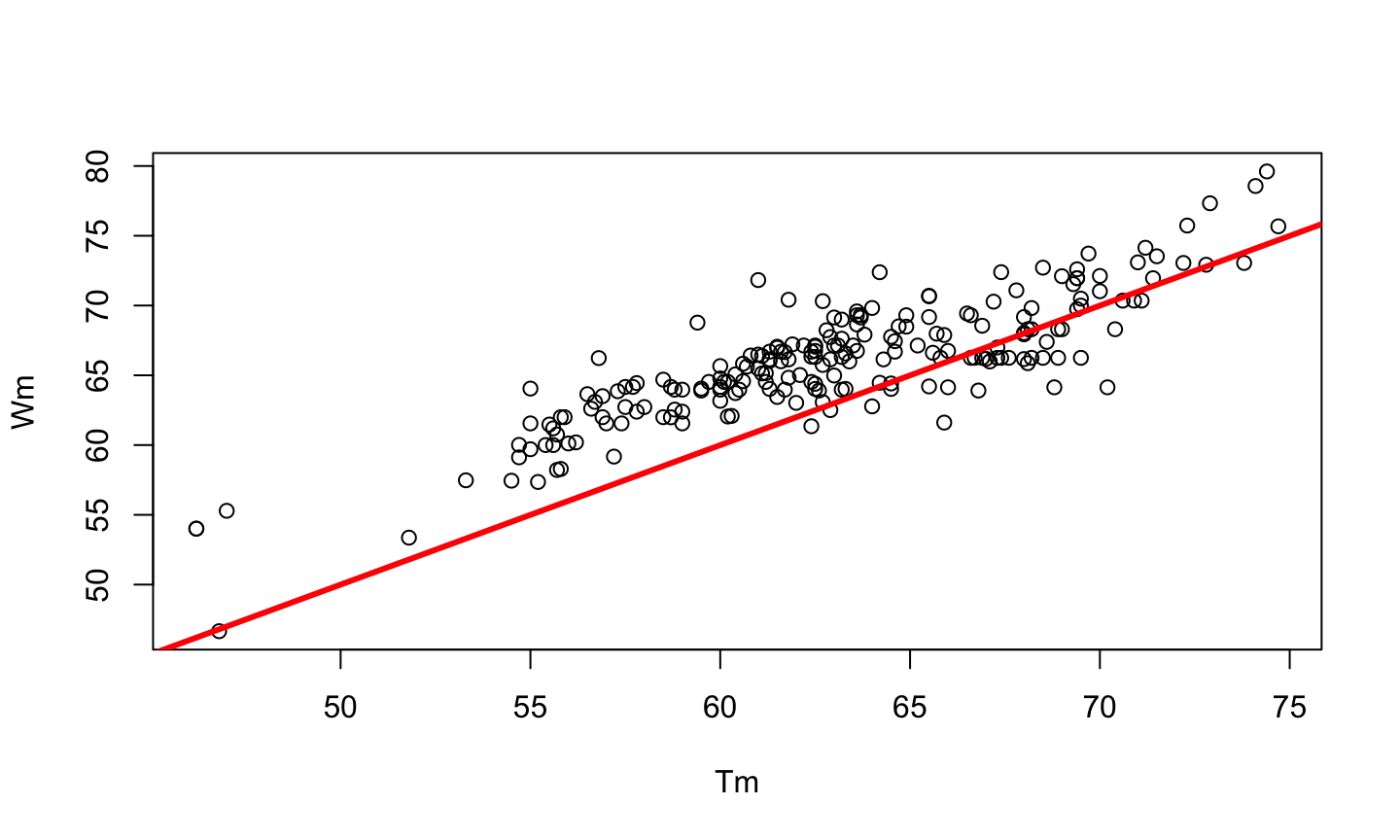
PCR is a method developed by Kary Mullis in the 1980s, used to synthesize millions of copies of a given DNA sequence

PCR is based on separate double-strand DNA using high temperatures, and complementing each DNA single-strand using a polymerase that works at these high temperatures

Taq DNA polymerase, which was isolated from Thermus aquaticus, can polymerize deoxynucleotide precursors (dNTPs) in a temperature range of 75-80^oC

Polymerase extends a pre-existing pairing, initially made by primers that bind spontaneously to specific sites on the DNA

A series of thermic cycles involving

• template DNA denaturation,
• primer annealing,
• and extension of the annealed primers by DNA polymerase

This three-step process is repeated 25-30 times Result: exponential accumulation of a specific fragment whose termini are defined by the 5’ ends of primers

1. Denaturation of the double-stranded target DNA by heating the sample to 90-95^oC
2. Temperature is lowered to about 5^oC below the melting temperature of the primer, assuring the specificity of the primer annealing and thus the specificity of the product
3. Raising the temperature of the sample to 70-73^oC, the optimal temperature for primer extension

Short DNA fragments with a defined sequence complementary to the target DNA that we want to detect and amplify

Each PCR assay requires the presence of template DNA, primers, nucleotides, and DNA polymerase

The primers in the reaction specify the exact DNA product to be amplified

The melting temperature (\(T_m\)) is the temperature of a DNA duplex in which half of the polymers are matched and half are not matched

The \(T_m\) shows the transition from double helical to random coil formation and it depends on the DNA GC base content, the cation concentration of the buffer and the DNA double strand length

It corresponds to the midpoint of the spectroscopic absorbance shift

\(T_m\) of an oligonucleotide is generally depend upon three major factors:

• Oligonucleotide concentration

• Salt concentration

• Oligonucleotide sequence

High DNA concentrations favor duplex formation and increase \(T_m\).

The \(T_m\) increases with higher ionic concentrations of the solvent due to the stabilizing effects that cations have on DNA duplex formation

More cations bind to duplex DNA than to the component single strands.

Different cations may have different effects on \(T_m.\) Most \(T_m\) research is done using \(Na^+\) as the primary cation; from a \(T_m\) standpoint, sodium, and potassium are equivalent

Divalent cations (such as \(Mg^{++}\)) also increase \(T_m\) but their effects are smaller than monovalent cations

Sequences with a higher fraction of G-C base pairs have a higher \(T_m\) than do AT-rich sequences

However, the \(T_m\) of an oligo is not simply the sum of AT and GC base content

Base stacking interactions must also be taken into account such that the actual specific sequence must be known to accurately predict \(T_m\)

The effects of neighboring bases as contributed through base stacking are called “nearest neighbor effects” which are accounted using experimentally determined thermodynamic parameters.

Different formulas have been developed, which can be classified into two groups:

• nucleotide composition
• position-dependent.

Nucleotide composition-based formulas depend on the GC-content, the number of base pairs, and the salt concentration

Position-dependent methods depend on parameters such as enthalpy (\(\Delta H^0\)), entropy (\(\Delta S^0\)), and Gibbs free energy (\(\Delta G^0\))

It is one of the simplest calculation methods, but it may not give the exact result for the calculation. \[T_m = 4(G+C)+2(A+T)\] The formula was originally applied to the hybridization of probes in 1 [mol/L] of NaCl and is an estimate of the denaturation temperature \((T_m)\):

This rule overestimates the \(T_m\) of long duplexes and gives reasonable results only in the range of 14-20 bp.

The linear relation between the GC content and the \(T_m\) was determined using absorbance shift analysis on sheared genomic DNA,

For a solvent containing 0.2 Molar of Na⁺, the melting temperature is \[T_m =69.3+0.41(\%GC)\] where \(T_m\) is in degrees Celsius. The measurement of the \(T_m\) is a way to determine the GC content of DNA

The \(T_m\) values are most simply measured by following the absorbance at 260 mM as a function of the temperature of the DNA solution and noting the midpoint of the hyperchromic rise. (Marmur & Doty, 1962).

Chester and Marshak added a term to account for DNA strand length (n in base pairs) to estimate primer \(T_m\):

\[T_m =69.3+0.41(\%GC)-\frac{650}{n}\]

It is easy to see that if the DNA molecule is big (for example, if \(n>10^6\)), then this formula gives the same result as Marmur and Doty

For ionic strength with a term for the \(Na^+\) concentration

\[T_m =81.5+16.6\log_{10}([Na^+])+0.41(\%GC)-\frac{b}{n}\]

Values between 500 and 750 have been used for \(b\) (a value that may increase with the ionic strength). Usually, the value \(b=500\) is used.

\[T_m =81.5+16.6\log_{10}\left(\frac{[Na^+]}{1.0+0.7[Na^+]}\right)+0.41(\%GC)-\frac{500}{n}\]

This formula includes these variables with the salt concentration term modified to extend the range to 1 M \(Na^+\), a concentration routinely employed to maximize hybridization rates on blots. (Wetmur, 1991)

These are methods that arise in the calculation of \(T_m\) using thermodynamic parameters (entropy \(\Delta S^0\), enthalpy \(\Delta H^0\), and Gibbs free energy \(\Delta G^0\)).

Measurements on a large number of oligomers revealed that thermodynamic values for helix-coil transition or DNA duplex melting did not depend only on base pair identity (A-T(U) or G-C).

Theoretical melting temperature is typically calculated assuming that the helix-coil transition is two-state, which is a justifiable assumption for small oligonucleotides.

SantaLucia, et al. suggest that the two-state model is capable of providing a reasonable approximation of melting temperature for duplexes with non-two-state transitions, but the applicability of the assumption obviously decreases as the size of the duplex under consideration increases.

single-strand + single-strand ⇆ double-strand

For self-complementary oligonucleotide duplexes, \(T_m\) is calculated from the predicted \(\Delta H^0\) and \(\Delta S^0\), and the total oligonucleotide concentration \(C_T\), by using the equation \[T_m=\frac{\Delta H^0}{\Delta S^0+R\ln (C_T)}\] where R is the Boltzmann’s gas constant (1.987[cal/Kmol]) and temperature is measured in Kelvin degrees. (SantaLucia, 1998) (Borer, Dengler, Tinoco, & Uhlenbeck, 1974)

\(\Delta G^0\) is the free energy. Each \(\Delta G^0\) term has enthalpic, \(\Delta H^0\), and entropic, \(\Delta S^0\) components. The \(\Delta G^0_{37}\) can also be calculated from \(\Delta H^0\) and \(\Delta S^0\) parameters by using the equation:

\[\Delta G^0_T=\Delta H^0(\text{total})-T\Delta S^0(\text{total})\] (SantaLucia, 1998)

\[\Delta G^0(\text{total})=\sum_i n_i\Delta G^0(i)+\Delta G^0(\text{init w/term G.C})\] \[+ \Delta G^0(\text{init w/term A.T})+\Delta G^0(\text{sym})\]

where \(\Delta G^0(i)\) are the standard free-energy changes for the 10 possible Watson-Crick Nearest-Neighbours (e.g., \(\Delta G^0(1)=\Delta G^0_{37}(AA/TT)\), \(\Delta G^0(2)=\Delta G^0_{37}(AT/TA)\) , …, etc.)

\(n_i\) is the number of occurrences of each Nearest-Neighbour, \(i\) and \(\Delta G^0(sym)\) equals +0.43[k cal/mol] if the duplex is self-complementary and zero if it is non-self-complementary